Summary: Equations of Ellipses in Standard Form

Thanks for your support!

 

• definition of an ellipse
• reflecting property of an ellipse
• equations of ellipses in standard form: foci on the $\,x$-axis
• (optional) getting an ellipse by stretching/shrinking a circle
• (optional) two useful transforms: reflection about the line $\,y = x\,$ and counterclockwise rotation by $\,90^\circ$
• equations of ellipses in standard form: foci on the $\,y$-axis

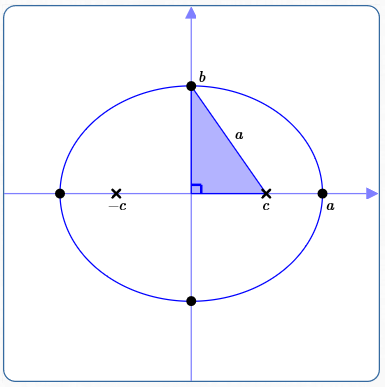
Foci on the $x$-axis Equation of Ellipse: $$\cssId{s12}{\frac{x^2}{\underset{\text{bigger}}{\underset{\uparrow}{a^2}}} + \frac{y^2}{b^2} = 1}$$ When the foci are on the $\color{red}{x}$-axis, the bigger number ($\,a^2 > b^2\,$) is beneath the $\,\color{red}{x}^2\,.$ Coordinates of foci: $\,(-c,0)\,$ and $\,(c,0)\,$

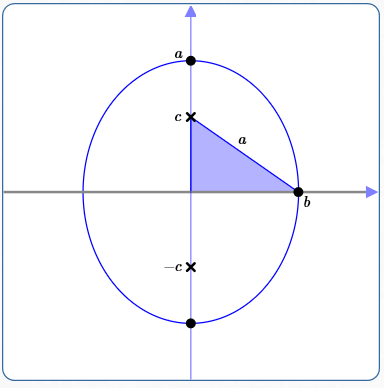
Foci on the $y$-axis Equation of Ellipse: $$\cssId{s19}{\frac{x^2}{b^2} + \frac{y^2}{\underset{\text{bigger}}{\underset{\uparrow}{a^2}}} = 1}$$ When the foci are on the $\color{red}{y}$-axis, the bigger number ($\,a^2 > b^2\,$) is beneath the $\,\color{red}{y}^2\,.$ Coordinates of foci: $\,(0,-c)\,$ and $\,(0,c)\,$

TIPS:

• The key to recognizing the equation of an ellipse with center at the origin and foci on either the $x$-axis or $y$-axis is this:
  • It has only $\,x^2\,,$ $\,y^2\,,$ and constant terms.
  • When the $\,x^2\,$ and $\,y^2\,$ terms are on the same side of the equation, then they must have the same sign.
• When the bigger number is beneath the $\,x^2\,$ term, then the foci are on the $\,x\,$-axis.
  When the bigger number is beneath the $\,y^2\,$ term, then the foci are on the $\,y\,$-axis.
  Look for the bigger denominator to tell you where the foci lie!
• Cut down on memorization—just use standard techniques to get the $x$ and $y$ intercepts.
  For example, in the equation $\displaystyle\,\frac{x^2}{a^2} + \frac{y^2}{b^2} = 1\,$:
  Let $\,y = 0\,$ to get the $x$-intercepts $\,a\,$ and $\,-a\,$:   $\displaystyle\,\frac{x^2}{a^2} = 1 \ \Rightarrow\ x^2 = a^2 \ \Rightarrow\ x = \pm a\,.$
  
  Let $\,x = 0\,$ to get the $y$-intercepts $\,b\,$ and $\,-b\,$:   $\displaystyle\,\frac{y^2}{b^2} = 1 \ \Rightarrow\ y^2 = b^2 \ \Rightarrow\ y = \pm b\,.$
• To find the foci: $\,c^2\,$ is always the bigger denominator minus the smaller denominator. $$\cssId{s39}{c^2 = \text{bigger denominator} - \text{smaller denominator}}$$

EXAMPLE: Graphing an Ellipse

Graph:   $100 - 4x^2 = 25y^2$

Initial thoughts:
There are only $\,x^2\,,$ $\,y^2\,$ and constant terms.
When the $\,x^2\,$ and $\,y^2\,$ terms are on the same side of the equation, they have the same sign.
It's an ellipse with center at the origin and foci on either the $x$-axis or $y$-axis!

Solution: $$ \begin{gather} \cssId{s48}{100 - 4x^2 = 25y^2}\cr\cr \cssId{s49}{4x^2 + 25y^2 = 100}\cr\cr \cssId{s50}{\frac{4x^2}{100} + \frac{25y^2}{100} = 1}\cr\cr \cssId{s51}{\frac{4x^2}{100}\cdot\frac{\frac 14}{\frac 14} + \frac{25y^2}{100}\cdot\frac{\frac 1{25}}{\frac 1{25}} = 1}\cr\cr \cssId{s52}{\frac{x^2}{25} + \frac{y^2}{4} = 1}\cr\cr \end{gather} $$ $x$-intercepts (set $\,y = 0\,$):   $\,x = \pm 5\,$ $y$-intercepts (set $\,x = 0\,$):   $\,y = \pm 2\,$ Foci: The bigger number is under the $\,x^2\,,$ so the foci are on the $x$-axis. $\,c^2 = 25 - 4 = 21\,$ $\,c = \pm\sqrt{21}$ $\,c\approx \pm 4.6\,$

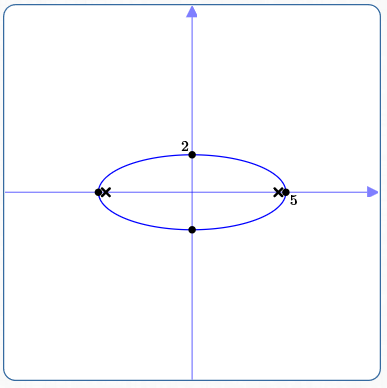
EXAMPLE: Finding the Equation of an Ellipse

Find the equation of the following ellipse:

• center at the origin
• major axis along the $y$-axis, with length $\,8\,$
• minor axis has length $\,6\,$

Also, find the coordinates of the foci.

Solution:

• Since the major axis is along the $y$-axis, the form of the equation is $$ \cssId{s68}{\frac{x^2}{b^2} + \frac{y^2}{a^2} = 1} $$
• $\,a\,$ is half the length of the major axis, which is $\,\frac{8}{2} = 4\,$
• $\,b\,$ is half the length of the minor axis, which is $\,\frac{6}{2} = 3\,$
  
• The equation is: $$ \begin{gather} \cssId{s72}{\frac{x^2}{3^2} + \frac{y^2}{4^2} = 1}\cr\cr \cssId{s73}{\frac{x^2}9 + \frac{y^2}{16} = 1} \end{gather} $$
• Foci:
  $\,c^2 = a^2 - b^2 = 16 - 9 = 7\,,$ so $\,c = \pm\sqrt{7}\,$
  Coordinates of foci: $\,(0,-\sqrt{7})\,$ and $\,(0,\sqrt{7})\,$

Master the ideas from this section
by practicing the exercise at the bottom of this page.

When you're done practicing, move on to:
Definition of a Hyperbola