Equations of Ellipses in Standard Form:
Foci on the $y$-axis

Thanks for your support!

 

Although it is much shorter, this derivation should look strikingly familiar to the earlier derivation.

Notice also that the variables $\,a\,,$ $\,b\,$ and $\,c\,$ have the same meaning as in the earlier derivation,
and the relationship between these three variables is the same.

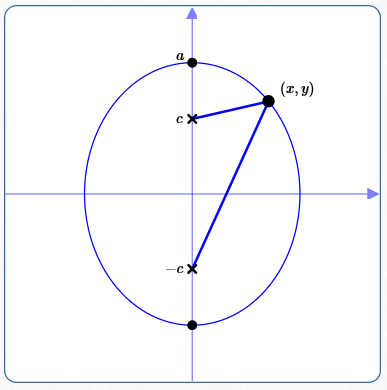
Position the ellipse: As shown at right, position an ellipse with center at the origin and foci (marked with ‘x’) on the $y$-axis. Notation ($\,c\,$ and $\,a\,$): Define: $c = \,$ the distance from the center (the origin) to each focus. Since $\,c\,$ is a distance, $\,c > 0\,.$ The coordinates of the foci are therefore $\,(0,c)\,$ and $\,(0,-c)\,.$ $a = \,$ the distance from the center to each vertex. Since $\,a\,$ is a distance, $\,a > 0\,.$ The coordinates of the vertices are therefore $\,(0,a)\,$ and $\,(0,-a)\,.$ Ellipse constant: The ellipse constant is the length of the major axis, which is $\,2a\,.$ Use the definition of ellipse on a typical point: Let $\,(x,y)\,$ be a typical point on the ellipse, so that the sum of its distances to the foci is the ellipse constant ($\,2a\,$). That is, $$ \cssId{s22}{\biggl( \text{distance from } (x,y) \text{ to } (0,-c) \biggr) + \biggl( \text{distance from } (x,y) \text{ to } (0,c) \biggr) = 2a} $$ Use the distance formula and make obvious simplications: $$ \begin{gather} \cssId{s24}{\sqrt{\bigl(x - 0\bigr)^2 + \bigl(y-(-c)\bigr)^2} + \sqrt{\bigl(x - 0\bigr)^2 + \bigl(y-c\bigr)^2} = 2a}\cr\cr \cssId{s25}{\sqrt{x^2 + (y+c)^2} + \sqrt{x^2 + (y-c)^2} = 2a} \end{gather} $$

• Isolate, undo, repeat:
  $$ \begin{alignat}{2} &\cssId{s27}{\sqrt{x^2 + (y+c)^2} = 2a - \sqrt{x^2 + (y-c)^2}}&\qquad&\cssId{s28}{\text{(isolate the first square root)}}\cr\cr &\cssId{s29}{x^2 + (y+c)^2 = 4a^2 - 4a\sqrt{x^2 + (y-c)^2} + x^2 + (y-c)^2}&&\cssId{s30}{\text{(square both sides and simplify)}}\cr\cr &\cssId{s31}{x^2 + y^2 + 2cy + c^2 = 4a^2 - 4a\sqrt{x^2 + (y-c)^2} + x^2 + y^2 - 2cy + c^2}&&\cssId{s32}{\text{(FOIL)}}\cr\cr &\cssId{s33}{2cy = 4a^2 - 4a\sqrt{x^2 + (y-c)^2} - 2cy} &&\cssId{s34}{\text{(addition property of equality)}}\cr\cr &\cssId{s35}{a\sqrt{x^2 + (y-c)^2} = a^2 - cy} &&\cssId{s36}{\text{(isolate square root term and divide by $\,4$)}}\cr\cr &\cssId{s37}{a^2 \bigl( x^2 + (y-c)^2 \bigr) = (a^2 - cy)^2} &&\cssId{s38}{\text{(square both sides and simplify)}}\cr\cr &\cssId{s39}{a^2 \bigl( x^2 + y^2 - 2cy + c^2 \bigr) = a^4 - 2a^2cy + c^2y^2} &&\cssId{s40}{\text{(FOIL)}}\cr\cr &\cssId{s41}{a^2x^2 + a^2y^2 - 2a^2cy + a^2c^2 = a^4 - 2a^2cy + c^2y^2}&&\cssId{s42}{\text{(distributive law)}}\cr\cr &\cssId{s43}{a^2x^2 + a^2y^2 - c^2y^2 = a^4 - a^2c^2} &&\cssId{s44}{\text{(addition property of equality and re-arrange)}}\cr\cr &\cssId{s45}{a^2x^2 + (a^2 - c^2)y^2 = a^2(a^2 - c^2)}&&\cssId{s46}{\text{(factor)}}\cr\cr \end{alignat} $$

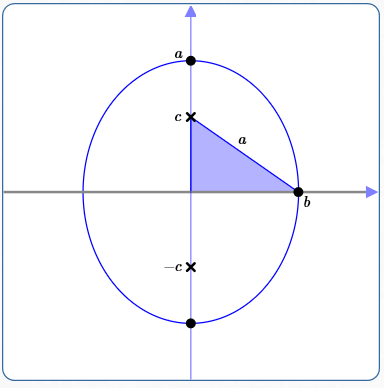
Define $\,b\,$; get relationship between $\,a\,,$ $\,b\,$ and $\,c\,$: As before, define $\,b\,$ as the distance from the center to an endpoint of the minor axis. Then, $\,c^2 + b^2 = a^2\,.$ Equivalently, $\,b^2 = a^2 - c^2\,$ and $\,c^2 = a^2 - b^2\,.$ Again, $\,a > b\,.$ Rewrite equation to eliminate $\,c\,,$ and simplify: $$ \begin{gather} \cssId{s53}{a^2x^2 + b^2y^2 = a^2b^2}\cr\cr \cssId{s54}{\frac{a^2x^2}{a^2b^2} + \frac{b^2y^2}{a^2b^2} = \frac{a^2b^2}{a^2b^2}}\cr\cr \cssId{s55}{\frac{x^2}{b^2} + \frac{y^2}{a^2} = 1} \end{gather} $$

Summary: The equation of an ellipse with center at the origin and foci along the $\,y$-axis is $$ \cssId{s58}{\frac{x^2}{b^2} + \frac{y^2}{a^2} = 1} $$ where: $a > b > 0$ The length of the major axis (which lies on the $\,y$-axis) is $\,2a\,.$ The length of the minor axis (which lies on the $\,x$-axis) is $\,2b\,.$ The foci are determined by solving the equation $\,c^2 = a^2 - b^2\,$ for $\,c\,.$ The coordinates of the foci are $\,(0,-c)\,$ and $\,(0,c)\,.$ Note: the foci are on the $\color{red}{y}$-axis, and the bigger number ($\,a^2 > b^2\,$) is beneath the $\,\color{red}{y}^2\,.$

Alternate Approach for Foci on the $y$-axis: Reflecting or Rotating

Once we have the equation for foci on the $x$-axis $\displaystyle\,\left(\frac{x^2}{a^2} +\frac{y^2}{b^2} = 1\right)\,,$
there are shorter and easier ways to get the equation for foci on the $y$-axis $\displaystyle\,\left(\frac{x^2}{b^2} +\frac{y^2}{a^2} = 1\right)\,.$
We didn't really have to go through this derivation again (although it's good practice).

Here are two ways.
For ease of reference, let $\,\cal G\,$ denote the graph of $\displaystyle\,\frac{x^2}{a^2} +\frac{y^2}{b^2} = 1\,.$
The two transformations used below are discussed in greater detail in this earlier optional lesson.

1. Reflect $\,\cal G\,$ about the line $\,y = x\,$:
   Reflection about the line $\,y = x\,$ switches the coordinates of a point:   $\,(x,y)\,$ moves to $\,(y,x)\,.$
   
   In an equation, this is accomplished by switching the variables $\,x\,$ and $\,y\,.$
   
   Switching $\,x\,$ and $\,y\,$ turns $\displaystyle\,\frac{x^2}{a^2} +\frac{y^2}{b^2} = 1\,$ into $\displaystyle\,\frac{y^2}{a^2} +\frac{x^2}{b^2} = 1\,.$
   
   Done!
2. Rotate $\,\cal G\,$ counterclockwise by $\,90^\circ\,$:
   Counterclockwise rotation by $\,90^\circ\,$ moves the point $\,(x,y)\,$ to the new point $\,(-y,x)\,.$
   
   In an equation, this is accomplished by:
   • replacing every $\,x\,$ by $\,y\,$
   • replacing every $\,y\,$ by $\,-x\,$
   
   These replacements turn $\displaystyle\,\frac{x^2}{a^2} +\frac{y^2}{b^2} = 1\,$ into: $$ \begin{gather} \cssId{s88}{\frac{y^2}{a^2} +\frac{(-x)^2}{b^2} = 1}\cr\cr \cssId{s89}{\frac{y^2}{a^2} + \frac{x^2}{b^2} = 1} \end{gather} $$ Easy!

Master the ideas from this section
by practicing the exercise at the bottom of this page.

When you're done practicing, move on to:
summary: equations of ellipses in standard form