The Square Root of a Negative Number

Square roots of negative numbers were introduced in a prior lesson:
Arithmetic with Complex Numbers in the Algebra II materials.
For your convenience, that material is repeated (and extended) here.

Firstly, recall some information from beginning algebra:

The Square Root of a Nonnegative Real Number

For a nonnegative real number $\,k\,$, $$ \cssId{s7}{\overbrace{\ \ \ \sqrt{k}\ \ \ }^{\text{the square root of $\,k\,$}}} \cssId{s8}{:=} \ \ \cssId{s9}{\text{the unique nonnegative real number which, when squared, equals $\,k\,$}} $$

(Note: when the distinction becomes important in higher-level mathematics, then $\,\sqrt{k}\,$ is called the principal square root of $\,k\,$.)

Thus, the number that gets to be called the square root of $\,k\,$ satisfies two properties:

it is nonnegative (not negative; i.e., greater than or equal to zero)
when squared, it gives $\,k\,$

For example, $\,\sqrt{9} = 3\,$ since the number $\,3\,$ satisfies these two properties:

$\,3\,$ is nonnegative
$\,3\,$, when squared, gives $\,9\,$: $\,3^2 = 9\,$

So, what is (say) $\,\sqrt{-4}\ $?
There does not exist a nonnegative real number which, when squared, equals $\,-4\ $.
Why not?
Every real number, when squared, is nonnegative: for all real numbers $\,x\,$, $\,x^2 \ge 0\,$.
Complex numbers to the rescue!

The Square Root of a Negative Number

Complex numbers allow us to compute the square root of negative numbers, like $\,\sqrt{-4}\ $.
Remember the key fact: $\,i:=\sqrt{-1}\,$, so that $\,i^2=-1\,$

THE SQUARE ROOT OF A NEGATIVE NUMBER

Let $\,p\,$ be a positive real number, so that $\,-p\,$ is a negative real number. Then: $$ \cssId{s31}{\overbrace{\ \ \ \sqrt{-p}\ \ \ }^{\text{the square root of negative $\,p\,$}}} \ \cssId{s32}{:=}\ \cssId{s33}{i\,\sqrt{\vphantom{h}p}} $$

(Note: when the distinction becomes important in higher-level mathematics, then $\,\sqrt{-p}\,$ is called the principal square root of $\,-p\,$.)

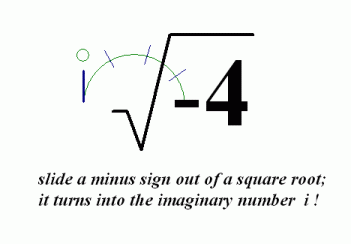
Observe that $\,i\sqrt{\vphantom{h}p}\,$, when squared, does indeed give $\,-p\ $: $$ \cssId{s36}{(i\sqrt{\vphantom{h}p})^2} \ \cssId{s37}{=\ i^2(\sqrt{\vphantom{h}p})^2} \ \cssId{s38}{=\ (-1)(p)} \ \cssId{s39}{=\ -p} $$

Some of my students like to think of it this way:
You can slide a minus sign out of a square root, and in the process, it turns into the imaginary number $\,i\,$!

sliding a minus sign out of a square root

Here are some examples:

$\sqrt{-4} = i\sqrt{4} = i2 = 2i\,$
It is conventional to write $\,2i\,$, not $\,i2\,$.
When there's no possible misinterpretation (see below), write a real number multiplier before the $\,i\,$.
$\sqrt{-5} = i\sqrt{5}$
In this situation, it's actually better to leave the $\,\sqrt{5}\,$ after the $\,i\,$.
Why? If you pull the $\,\sqrt{5}\,$ to the front, it can lead to a misinterpretation, as follows:
The numbers $\,\sqrt{5}i\,$ and $\,\sqrt{5i}\,$ are different numbers—look carefully!
In $\ \sqrt{5}i\ $, the $\,i\,$ is outside the square root.
In $\ \sqrt{5i}\ $, the $\,i\,$ is inside the square root.
Unless you look carefully, however, you might mistake one of these for the other.
By writing $\,i\sqrt{5}\,$, you eliminate any possible confusion.

TWO DIFFERENT QUESTIONS; TWO DIFFERENT ANSWERS

Recall these two different questions, with two different answers:

What is $\,\sqrt{4}\,$?
Answer: By definition, $\,\sqrt{4} = 2\,$.
The number $\ \sqrt{4}\ $ is the nonnegative number which, when squared, gives $\,4\,$.
What are the solutions to the equation $\,x^2 = 4\,$?
Answer: $\,x= \pm\ 2\,$
There are two different real numbers which, when squared, give $\,4\,$.

Similarly, there are two different questions involving complex numbers, with two different answers:

What is $\,\sqrt{-4}\,$?
Answer: $\sqrt{-4} = 2i\,$
What are the (complex) solutions to the equation $\,x^2 = -4\,$?
Answer: $\,x=\pm \sqrt{-4} = \pm\ 2i\,$
There are two different complex numbers which, when squared, give $\,-4\,$.
Verifying the second solution: $\, \cssId{s74}{(-2i)(-2i)} \cssId{s75}{= 4i^2} \cssId{s76}{= 4(-1)} \cssId{s77}{= -4} $

Square Roots of Products

The following statement is true: for all nonnegative real numbers $\,a\,$ and $\,b\,$, $\,\sqrt{ab} = \sqrt{a}\sqrt{b}\ $.
For nonnegative numbers, the square root of a product is the product of the square roots.

Does this property work for negative numbers, too?
The answer is NO, as shown next.

Certainly, anything called ‘the square root of $\,-4\,$’ must have the property that, when squared, it equals $\,-4\ $.
Unfortunately, the following incorrect reasoning gives the square as $\,4\,$, not $\,-4\,$: $$ \cssId{s87}{\text{? ? ? ? }}\ \ \ \ \cssId{s88}{(\sqrt{-4})^2} \ \ \cssId{s89}{=\ \ \sqrt{-4}\sqrt{-4}} \cssId{s90}{\overbrace{\ \ =\ \ }^{\text{this is the mistake}}}\ \ \cssId{s91}{\sqrt{(-4)(-4)}}\ \ \cssId{s92}{=\ \ \sqrt{16}}\ \ \cssId{s93}{=\ \ 4} \ \ \ \ \cssId{s94}{\text{? ? ? ? }} $$ Here is the correct approach: $$ \cssId{s96}{(\sqrt{-4})^2} \ \ \cssId{s97}{=\ \ \sqrt{-4}\sqrt{-4}}\ \ \cssId{s98}{=\ \ i\sqrt{4}\ i\sqrt{4}} \ \ \cssId{s99}{=\ \ i^2(\sqrt{4})^2} \ \ \cssId{s100}{=\ \ (-1)(4)} \ \ \cssId{s101}{=\ \ -4} $$

Similarly, $\,\sqrt{-5}\sqrt{-3}\,$ is NOT equal to $\,\sqrt{(-5)(-3)}\,$.
Instead, here is the correct simplication: $$ \cssId{s104}{\sqrt{-5}\sqrt{-3}} \ \ \cssId{s105}{=\ \ i\sqrt{5}\ i\sqrt{3}} \ \ \cssId{s106}{=\ \ i^2\sqrt{5}\sqrt{3}} \ \ \cssId{s107}{=\ \ (-1)\sqrt{(5)(3)}} \ \ \cssId{s108}{=\ \ -\sqrt{15}} $$ Be careful about this!

Master the ideas from this section
by practicing the exercise at the bottom of this page.

When you're done practicing, move on to:
the complex conjugate