 Finding Slant Asymptotes
Finding Slant Asymptotes
Drop an object from far above the Earth, and it falls faster and faster—for a while.
Indeed, since acceleration due to gravity is about $\frac{9.8 \text{ meters per second}}{\text{second}}\,,$ its speed initially increases by about 9.8 m/sec every second.
But, it doesn't keep going faster and faster. Due to frictional forces, it settles on a so-called ‘terminal velocity’.
If you model distance traveled versus time mathematically, you'll be looking at a slant asymptote.
Functions can ‘get big’ in different ways. When they ‘get big’ by looking more and more like a ‘slanted’ line (i.e., not horizontal and not vertical), then the function is said to have a slant asymptote.
Slant asymptotes are also called oblique asymptotes.
As discussed in Introduction to Asymptotes, an asymptote is a curve (usually a line) that another curve gets arbitrarily close to as $\,x\,$ approaches $\,+\infty\,,$ $\,-\infty\,,$ or a finite number.
Here's how this general definition is ‘specialized’ to get a slant asymptote:
A ‘slant asymptote’ is a non-vertical, non-horizontal line that another curve gets arbitrarily close to as $\,x\,$ approaches $\,+\infty\,$ or $\,-\infty\,.$ (See image below.)
What's the key to a slant asymptote situation?
The outputs are behaving more and more like $\,y = mx + b\,$ for $\,m\ne 0\,$ as inputs get arbitrarily large (big and positive, or big and negative).
This section gives a precise discussion of slant asymptotes, as well as conditions under which a rational function has a slant asymptote.
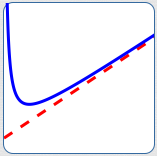
The red line is a slant asymptote for the blue curve.
As $\,x\rightarrow\infty\,,$ the blue curve approaches the red line.
Let $\,f\,$ be a nonlinear function. That is, $\,f(x)\,$ is not of the form $\,f(x) = cx + d\,$ for any real numbers $\,c\,$ and $\,d\,.$
Let $\,m \ne 0\,.$ The line $\,y = mx + b\,$ is a slant asymptote for $\,f\,$ if and only if at least one of the following conditions holds:
- As $\,x\rightarrow\infty\,,$ $\,f(x) - (mx + b) \rightarrow 0\,$
- As $\,x\rightarrow -\infty\,,$ $\,f(x) - (mx + b) \rightarrow 0\,$
That is, the vertical distance between the line $\,y = mx + b\,$ and the graph of $\,f\,$ approaches zero as $\,x\rightarrow\infty\,$ or $\,x\rightarrow -\infty\,$ (or both).
- A function can have at most two slant asymptotes. It can approach one line as $\,x\rightarrow\infty\,,$ and a different line as $\,x\rightarrow -\infty\,.$
- A function can have a slant asymptote and a horizontal asymptote. For example, it might approach a horizontal line as $\,x\rightarrow\infty\,,$ and a slanted line as $\,x\rightarrow -\infty\,.$
Example
A Non-Rational Function With a Slant Asymptote
Let $\,f(x) = 3x + \text{e}^{-x}\,$ (the blue curve below).
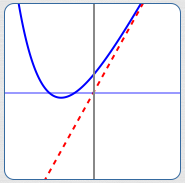
As $\,x\rightarrow \infty\,,$ we have $\,\text{e}^{-x}\rightarrow 0\,,$ so the outputs from $\,f\,$ are looking more and more like $\,3x\,.$
More precisely, as $\,x\rightarrow \infty\,,$ we have:
$$ \begin{gather} \cssId{s41}{f(x) - 3x \ = \ (3x + \text{e}^{-x}) - 3x \ = \ \text{e}^{-x}}\cr \cssId{s42}{\text{and}}\cr \cssId{s43}{\text{e}^{-x}\rightarrow 0} \end{gather} $$Thus, $\,y = 3x\,$ (the red line above) is a slant asymptote for $\,f\,.$
Example
A Rational Function With a Slant Asymptote: Degree of Numerator is Exactly One Greater Than Degree of Denominator
Let $\displaystyle\,R(x) = \frac{x^2 - 4x - 5}{x - 3}\,.$
When $\,x\,$ is big, the outputs from $\,R(x)\,$ look like $\,\frac{\text{big}}{\text{big}}\,,$ which is not very useful. As usual, we will rename the function to better understand what happens when $\,x\,$ is big.
The key is to do a long division:
| $x$ | $-$ | $1$ | |||
| $x-3$ | $x^2$ | $-$ | $4x$ | $-$ | $5$ |
| $-(x^2$ | $-$ | $3x$ | $)$ | ||
| $-x$ | $-$ | $5$ | |||
| $-($ | $-x$ | $+$ | $3)$ | ||
| $-8$ | |||||
Thus:
$$ \begin{align} \cssId{s54}{R(x)}\ &\cssId{s55}{= \frac{x^2 - 4x - 5}{x - 3}}\cr &\cssId{s56}{=\ x - 1 \ \ \ + \overbrace{\left(\frac{-8}{x-3}\right)}^{\text{this part tends to zero}}} \end{align} $$Note that as $\,x\rightarrow\pm\infty\,,$ $\displaystyle\,\frac{-8}{x-3}\rightarrow 0\,.$
Therefore, as inputs get big, the function is looking more and more like the line $\,y = x - 1\,.$ Thus, $\,y = x - 1\,$ is a slant asymptote for the function $\,R\,.$
The graph of the function $\,\color{blue}{R}\,$ (in blue), together with its slant asymptote $\,\color{red}{y = x - 1}\,$ (in red), is shown below.
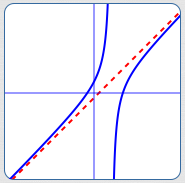
When we were doing the long division of $\,x^2 - 4x - 5\,$ by $\,x - 3\,$ up above, what caused the $\,x = x^1\,$ to appear in the quotient $\,x - 1\,$?
It was the fact that the degree of the numerator is one more than the degree of the denominator! This is the key ingredient for determining if a rational function has a slant asymptote. (There are a couple other technical requirements, which are stated below.)
In summary, we have:
Let $\,N(x)\,$ and $\,D(x)\,$ be polynomials, so that $\displaystyle\,R(x) = \frac{N(x)}{D(x)}\,$ is a rational function.
The function $\,R\,$ has a slant asymptote when the following conditions are met:
-
$\deg{N(x)} = \deg{D(x)} + 1$
(The degree of the numerator is exactly one more than the degree of the denominator.) -
$\deg{N(x)} \ge 2$
(The numerator is at least quadratic.) - When dividing $\,D(x)\,$ into $\,N(x)\,,$ the remainder is not zero.
Why Do We Require That $\deg{N(x)}\ge 2\,$?
Notice that $\displaystyle \,y = \frac{x-1}{3}\,$ is a rational function, since both numerator and denominator are polynomials.
The degree of the numerator is $\,1\,.$ The degree of the denominator is $\,0\,.$ Thus, the degree of the numerator is exactly one more than the degree of the denominator.
However, this is just the linear function $\,y = \frac 13x - \frac 13\,,$ which does not have a slant asymptote.
Why Do We Require The Remainder To Be Nonzero?
Notice that $\displaystyle \,y = \frac{x^2-1}{x-1}\,$ is a rational function, and the degree of the numerator is exactly one more than the degree of the denominator.
However, in this case $\,x - 1\,$ goes into $\,x^2 - 1\,$ evenly (the remainder is zero):
$$ \begin{align} y \ \ &\cssId{s87}{= \frac{x^2-1}{x-1}}\cr\cr &\cssId{s88}{= \frac{(x-1)(x+1)}{x-1}}\cr\cr &\cssId{s89}{= x + 1\,,} \ \ \cssId{s90}{\text{for $\,x\ne 1\,$}} \end{align} $$This function graphs as a line that is punctured at $\,x = 1\,.$ It does not have a slant asymptote.