Introduction to Polynomials

First, review the Introduction to Polynomials lesson in the Algebra II curriculum to make sure you've mastered basic polynomial concepts and terminology.

For example, you should know:

The Definition of a Polynomial

A polynomial is a finite sum of terms, each of the form $\,ax^k\,,$ where $\,a\,$ is a real number, and $\,k\,$ is a nonnegative integer. That is: $\,k\in \{0,1,2,3,\ldots\}$

How to Put a Polynomial in Standard Form

The standard form of a polynomial is: $$ \cssId{s11}{a_nx^n + a_{n-1}x^{n-1} + \cdots + a_1 + a_0} $$ Here, $\,n\,$ denotes the highest power to which $\,x\,$ is raised.

Thus, in standard form, the highest power term is listed first, and subsequent powers are listed in decreasing order.

How to Determine a Polynomial's Degree

The degree of the polynomial is the highest power to which $\,x\,$ is raised.

How to Determine a Polynomial's Leading Coefficient

The coefficient of the highest power term is called the leading coefficient of the polynomial.

When a polynomial is written in standard form, the leading coefficient actually leads the polynomial.

For example, $$P(x) = -3x + 11 - 5x^7 + 9x^2$$ is a polynomial.

Its standard form is: $$P(x) = -5x^7 + 9x^2 - 3x + 11$$

Its degree is $\,7\,.$ Its leading coefficient is $\,-5\,.$

For a more thorough review and practice exercises, study Introduction to Polynomials in the Algebra II curriculum.

Relationship between the Zeros and Factors of a Polynomial

There is a beautiful relationship between the zeros and factors of a polynomial, which is explored in the next section. In preparation, the concepts of zero and factor are reviewed below.

Zeros (Roots) of Functions

It would be reasonable to guess that a zero of a function has something to do with the number $\,0\,.$ It does!

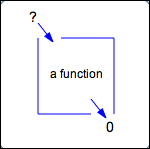
function box view of the zero of a function

What input (or inputs) must be dropped in the top of a function box, to get zero out the bottom?

A zero of a function is an input whose corresponding output is zero.

Example (Finding the Zeros of a Function)

Consider the function $\,f(x) = x^2 - 2x + 1\,.$ Recall that $\,f(x)\,$ represents the output from the function $\,f\,$ when the input is $\,x\,.$

What value(s) of $\,x\,$ make $\,f(x)\,$ equal to $\,0\,$?
$f(x) = 0$	Set the output, $\,f(x)\,,$ equal to zero.
$x^2 - 2x + 1 = 0$	Substitution: $\,f(x)\,$ is $\,x^2 - 2x + 1\,$
$(x-1)^2 = 0$	Factor: $\,x^2 - 2x + 1 = (x-1)^2$
$x-1 = 0$	$z^2 = 0\,$ if and only if $\,z = 0$
$x = 1$	Add $\,1\,$ to both sides.

In this example, there is only one input, $\,x = 1\,,$ for which the output is zero.

Check:

$$\cssId{s54}{f(1) = 1^2 - 2(1) + 1 = 0}$$

Here, $\,1\,$ is the only zero of the function $\,f\,.$

A zero of a function is also called a root of the function.

Initially, you might find yourself a bit uncomfortable saying ‘$\,1\,$ is a zero’. It almost sounds like you're saying ‘$\,1 = 0\,$’, which is of course false. The word ‘a’ is critically important in the sentence!

When you say:	$\,1\,$ is a zero
You're saying:	$\,1\,$ is an input whose corresponding output is zero

Example (Zeros of Non-Polynomials)

You can talk about zeros of any function—not just polynomials.

For example, the number $\,2\,$ is a zero of $\,f(x) = \sqrt{x - 2}\,$ since $\,f(2) = \sqrt{2-2} = 0\,.$

The function $\,f(x) = {\text{e}}^x\,$ doesn't have any zeros, since $\,{\text{e}}^x\,$ is always strictly greater than zero.

Equivalent Characterizations of a Zero of a Function

There are several equivalent ways to think about zeros of functions:

ZEROS (ROOTS) OF FUNCTIONS

A zero (or root) of a function is an input, whose corresponding output is zero.

Let $\,f\,$ be a function, and let $\,c\,$ be an input to $\,f\,.$

Then, the following are equivalent:

$c\,$ is a zero of $\,f$
$c\,$ is a root of $\,f$
$c\,$ is an input, with corresponding output $\,0$
$f(c) = 0$
the point $\,(c,0)\,$ lies on the graph of $\,f\,$
$f\,$ has an $x$-intercept equal to $\,c$
the graph of $\,f\,$ crosses the $x$-axis at $\,c\,$
$x = c\,$ is a solution of the equation $\,f(x) = 0$

In particular, observe that zeros are easy to spot if you have the graph of a function: they are the $x$-intercepts.

Review: Factors of a (Whole) Number

Early on, you learned that the factors of (say) $\,27\,$ are numbers that go into $\,27\,$ evenly (with no remainder). ‘No remainder’ means the same thing as a remainder of zero.

For example, $\,3\,$ is a factor of $\,27\,.$ Why? Because it goes into $\,27\,$ nine times: $27 = 3\cdot 9$

The number (say) $\,11\,$ is not a factor of $\,27\,.$ Why not? Because there is a nonzero remainder—it goes in twice, with a remainder of $\,5\,.$ That is: $\,27 = 2\cdot 11 + 5$

Note that when you write $\,27 = 3\cdot 9\,,$ the number $\,27\,$ has been expressed as a product (the last operation is multiplication). However, when you write $\,27 = 2\cdot 11 + 5\,,$ the number $\,27\,$ has been expressed as a sum (the last operation is addition).

Key Idea: The factors of a number can be used to express the number as a product. Indeed, factoring means to take something and express it as a product.

Factors of a Polynomial

In a similar way, we can talk about factors of a polynomial. These are expressions that go into the polynomial evenly (with no remainder). (Note: long division of polynomials is covered in a future section.)

For example, let: $$P(x) = x^2 + x - 6$$

The expression $\,x-2\,$ is a factor of $\,P(x)\,.$ Why? Because it goes into $\,P(x)\,$ evenly: it goes in $\,x + 3\,$ times.

That is: $$x^2 + x - 6 = (x-2)(x+3)$$

Check by multiplying out:

$$\begin{align} &(x-2)(x+3)\cr &\qquad = x^2 + 3x - 2x - 6\cr &\qquad = x^2 + x - 6 \end{align} $$

The expression (say) $\,x+5\,$ is not a factor of $\,P(x)\,.$ Why not? Because when $\,x+5\,$ is divided into $\,P(x)\,,$ there is a nonzero remainder.

Indeed, long division of polynomials shows that $\,x+5\,$ goes into $\,P(x)\,$ $\,x-4\,$ times, with a remainder of $\,14\,.$ That is:

$$\cssId{s124}{x^2 + x - 6 = (x+5)(x-4) + 14}$$

Check by multiplying out and adding:

$$ \begin{align} &(x+5)(x-4) + 14\cr &\qquad = x^2 - 4x + 5x - 20 + 14\cr &\qquad = x^2 + x - 6 \end{align} $$

Note that when you write $\,P(x) = (x-2)(x+3)\,,$ the polynomial $\,P(x)\,$ has been expressed as a product (the last operation is multiplication).

However, when you write $\,P(x) = (x+5)(x-4) + 14\,,$ the polynomial $\,P(x)\,$ has been expressed as a sum (the last operation is addition).

Key Idea: The factors of a polynomial can be used to express the polynomial as a product.

Looking Ahead to the Next Section...

Expressions have lots of different names, and different names are good for different things.

When you write $\,P(x) = x^2 + x - 6\,,$ it's not the least bit clear (at least to this author) what value(s) of $\,x\,$ make the output zero. What number, when squared, then added to itself, then with $\,6\,$ subtracted, gives zero? Not a clue!

However, when you write $\,P(x) = (x-2)(x+3)\,,$ the zeros of $\,P\,$ jump out at you!

The number $\,2\,$ is a zero:

$$ \begin{align} P(2) &= (2-2)(2+3)\cr &= 0\cdot 5\cr &= 0 \end{align} $$

The number $\,-3\,$ is a zero:

$$ \begin{align} P(-3) &= (-3-2)(-3+3)\cr &= -5\cdot 0\cr &= 0 \end{align} $$

Returning to the other name (just for fun):

$$ \cssId{s146}{ \begin{align} P(2) &= 2^2 + 2 - 6\cr &= 4 + 2 - 6\cr &= 0 \end{align}} $$ $$ \cssId{s147}{ \begin{align} P(-3) &= (-3)^2 + (-3) - 6\cr &= 9 - 3 - 6\cr &= 0 \end{align}} $$

You may have noticed the beautiful relationship between zeros and factors:

$x-\color{blue}{2}$ is a factor;
$\color{blue}{2}\,$ is a zero

$x + 3 = x-(\color{blue}{-3})$ is a factor;
$\color{blue}{-3}\,$ is a zero

This observation is formalized in the next section!