Partial Fraction Expansion: Irreducible Quadratic Factors

Partial Fraction Expansion (PFE) renames a fraction of polynomials (i.e., a rational function), using smaller, simpler ‘pieces’.

This is the third of three sections covering PFE:

The first section introduces PFE, reviews all needed concepts, and presents a simple example (distinct linear factors).

The second section gives the complete ‘how-to’ of PFE and a detailed example (repeated linear factor).

This current section presents two detailed examples, both of which illustrate how to handle irreducible quadratic factors:

• PFE with an Initial Long Division, Factoring a Difference of Cubes, and Distinct Irreducible Quadratic Factor
• PFE with a Repeated Irreducible Quadratic Factor

The steps indicated in the examples below follow the summary of the previous section.

Example:  PFE with an Initial Long Division, Factoring a Difference of Cubes, and Distinct Irreducible Quadratic Factor

Step 1 (Check Degree of Numerator)

The degree of the numerator ($\,4\,$) is not less than the degree of the denominator ($\,3\,$). Therefore, an initial long division is required, which yields:

In Steps 2–4 below, PFE is applied to the remainder term, $\,\color{green}{\frac{4x^2-3x+2}{x^3-1}}\,.$ In Step 5, we'll need to be sure not to forget the $\,\color{red}{2x}\,$!

Step 2 (Factor Denominator)

The denominator, $\,x^3 - 1\,,$ must be factored into linear factors and irreducible quadratics. The difference of cubes formula comes to the rescue:

The factor $\,x-1\,$ is a distinct linear factor. The factor $\,x^2 + x + 1\,$ is a distinct irreducible quadratic factor, since its discriminant is negative:

Step 3 (Form of PFE, With Unknown Constants)

The distinct linear factor, $\,x-1\,,$ gives rise to a single term in the PFE, with a single unknown: say, $\,\frac{A}{x-1}\,.$

The distinct irreducible quadratic factor, $\,x^2 + x +1\,,$ gives rise to a single term in the PFE, with two unknowns: say, $\,\frac{Bx+C}{x^2+x+1}\,.$

Of course, names other than $\,A\,,$ $\,B\,,$ and $\,C\,$ could be used for the unknown constants.

The form of the PFE for $\,\frac{4x^2-3x + 2}{x^3-1}\,$ is therefore:

Step 4 (Clear Fractions; Solve For Unknown Constants)

Clear fractions in ($\,\dagger\,$) by multiplying both sides of the equation by $\,(x-1)(x^2+x+1)\,.$ This gives:

It's always easy to solve for an unknown corresponding to a distinct linear factor. Always get these easy unknowns first!

In this example, the distinct linear factor $\,x-1\,$ corresponds to the term $\,\frac{A}{x-1}\,$ with unknown $\,A\,.$

So, $\,A\,$ is easy to find:  let $\,x = 1\,$ in ($\,\ddagger\,$), since this makes $\,x-1\,$ equal to zero:

It now remains to solve for $\,B\,$ and $\,C\,.$

In what follows, it's important that when you look at $\,(Bx+C)(x-1)\,,$ you actually ‘see’ (in your mind's eye) the multiplied-out version:

(If necessary, multiply it out on paper.)

Thus, $\,B\,$ appears in the coefficients of both $\,x^2\,$ and $\,x\,$ terms. Also, $\,C\,$ appears in the coefficient of an $\,x\,$ term, and in a constant term.

There are choices for how to solve for $\,B\,$ and $\,C\,,$ and some are much better than others!

A few different approaches are shown below—there are many more. It's your job to discover the easy ways! Definitely try to avoid approaches that require solving a system of equations, because these are more error-prone.

The equation that the unknowns ($\,A\,,$ $\,B\,$ and $\,C\,$) must satisfy is repeated here for your convenience:

Here's the same equation with the right-hand side in standard form, so it's easier to compare the coefficients of each term type. In practice, however, try to ‘see’ all this from ($\,\ddagger\,$), without multiplying it out.

Recall that it has already been determined that $\,A = 1\,.$

Of course, all correct approaches will lead to the same results. (The author prefers the first approach.)

Thus, we have:

Step 5 (Summarize Results; Spot-Check)

Combine the results from Step 1 and Step 4 to get:

Spot-check with $\,x = 0\,$ to gain confidence in the result:

Example:  PFE With a Repeated Irreducible Quadratic Factor

(The following solution is much more compact than the prior example.)

Step 1 (Check Degree of Numerator)

The degree of the numerator ($\,2\,$) is less than the degree of the denominator ($\,4\,$). Check!

Step 2 (Factor Denominator)

The denominator is a ‘fake quadratic’ (also called a ‘pseudo-quadratic’):

The quadratic $\,x^2 + 3\,$ is irreducible. Why? Use any of the following equivalent characterizations:

• The discriminant is negative:
  $$ \begin{align} b^2 - 4ac &= 0^2 - 4(1)(3)\cr &= -12\cr &\lt 0 \end{align} $$
• There are no real number solutions to $\,x^2 + 3 = 0$
• The graph of $\,y = x^2 + 3\,$ never crosses the $x$-axis

Thus, $\,(x^2 + 3)^2\,$ is a repeated irreducible quadratic factor.

Step 3 (Form of PFE, with Unknown Constants)

The repeated irreducible quadratic factor, $\,(x^2 + 3)^\color{red}{2}\,,$ gives rise to two terms in the PFE, each with two unknowns:

Step 4 (Clear Fractions; Solve for Unknown Constants)

Clear fractions in ($\,\dagger\,$) to get:

Equate $\,x^3\,$ coefficients in ($\,\ddagger\,$) to get $\,A = 0\,.$

Equate $\,x^2\,$ coefficients in ($\,\ddagger\,$) to get $\,B = 3\,.$

Equate $\,x\,$ coefficients in ($\,\ddagger\,$) to get:

Equate constants in ($\,\ddagger\,$) to get:

Step 5 (Summarize Results; Spot-Check)

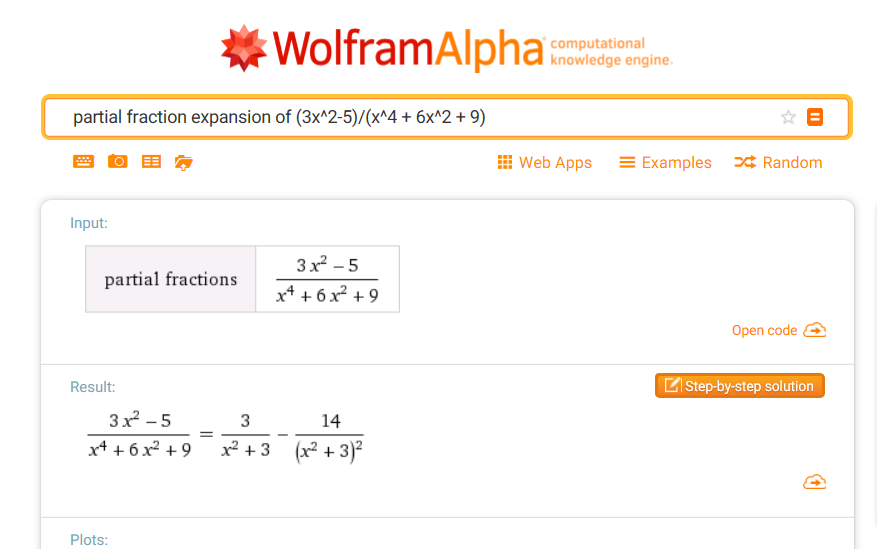
Thus:

Spot-check with $\,x = 0\,$ to gain confidence in the result:

Or, zip up to WolframAlpha for the ultimate confidence booster: