Graphing Tools: Vertical and Horizontal Scaling (Part 2)

(This page is Part 2. Click here for Part 1.)

Ideas Regarding Horizontal Scaling (Stretching/Shrinking)

• Points on the graph of $\,y=f(x)\,$ are of the form $\,\bigl(x,f(x)\bigr)\,.$

  Points on the graph of $\,y=f(3x)\,$ are of the form $\,\bigl(x,f(3x)\bigr)\,.$

• How can we locate these desired points $\,\bigl(x,f(3x)\bigr)\,$?

  

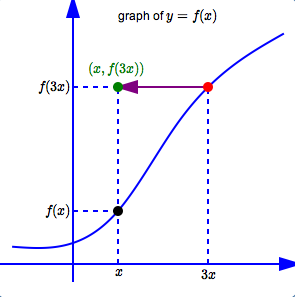
  First, go to the point $\,\color{red}{\bigl(3x\,,\,f(3x)\bigr)}\,$ on the graph of $\,\color{red}{y=f(x)\,.}$ This point has the $y$-value that we want, but it has the wrong $x$-value. The $x$-value of this point is $\,3x\,,$ but the desired $x$-value is just $\,x\,.$

  Thus, the current $\,\color{purple}{x}$-value must be divided by $\,\color{purple}{3}\,$; the $\,\color{purple}{y}$-value remains the same. This gives the desired point $\,\color{green}{\bigl(x,f(3x)\bigr)}\,.$

  Thus, the graph of $\,y=f(3x)\,$ is the same as the graph of $\,y=f(x)\,,$ except that the $x$-values have been divided by $\,3\,$ (not multiplied by $\,3\,,$ which you might expect).

  Notice that dividing the $x$-values by $\,3\,$ moves them closer to the $y$-axis; this is called a horizontal shrink.

• Transformations involving $\,x\,$ do NOT work the way you would expect them to work! They are counter-intuitive—they are against your intuition.

• Here is the thought process you should use when you are given the graph of $\,y=f(x)\,$ and asked about the graph of $\,y=f(3x)\,$:

interpretation of new equation:

• Replacing every $\,x\,$ by $\,3x\,$ in an equation causes the $x$-values in the graph to be DIVIDED by $\,3\,.$

Summary of Horizontal Scaling

Let $\,k\gt 1\,.$ Start with the equation $\,y=f(x)\,.$ Replace every $\,x\,$ by $\,kx\,$ to give the new equation $\,y=f(kx)\,.$

This causes the $x$-values on the graph to be DIVIDED by $\,k\,,$ which moves the points closer to the $y$-axis. This is called a horizontal shrink.

A point $\,(a,b)\,$ on the graph of $\,y=f(x)\,$ moves to a point $\,(\frac{a}{k},b)\,$ on the graph of $\,y=f(kx)\,.$

Additionally: Let $\,k\gt 1\,.$ Start with the equation $\,y=f(x)\,.$ Replace every $\,x\,$ by $\,\frac{x}{k}\,$ to give the new equation $\,y=f(\frac{x}{k})\,.$

This causes the $x$-values on the graph to be MULTIPLIED by $\,k\,,$ which moves the points farther away from the $y$-axis. This is called a horizontal stretch.

A point $\,(a,b)\,$ on the graph of $\,y=f(x)\,$ moves to a point $\,(ka,b)\,$ on the graph of $\,y=f(\frac{x}{k})\,.$

This transformation type is formally called horizontal scaling (stretching/shrinking).

Different Words Used to Talk About Transformations Involving $\,y\,$ and $\,x\,$

Notice that different words are used when talking about transformations involving $\,y\,,$ and transformations involving $\,x\,.$

For transformations involving $\,y\,$ (that is, transformations that change the $y$-values of the points), we say:

DO THIS to the previous $\,y$-value.

For transformations involving $\,x\,$ (that is, transformations that change the $x$-values of the points), we say:

REPLACE the previous $x$-values by $\ldots$

Make Sure You See The Difference!

Vertical Scaling:
going from  $\,y=f(x)\,$  to  $\,y = kf(x)\,$  for  $\,k\gt 0$

Horizontal Scaling:
going from  $\,y = f(x)\,$  to  $\,y = f(kx)\,$  for  $\,k\gt 0$

Make sure you see the difference between (say) $\,y = 3f(x)\,$ and $\,y = f(3x)\,$!

In the case of $\,y = 3f(x)\,,$ the $\,3\,$ is ‘on the outside’; we're dropping $\,x\,$ in the $\,f\,$ box, getting the corresponding output, and then multiplying by $\,3\,.$ This is a vertical stretch.

In the case of $\,y = f(3x)\,,$ the $\,3\,$ is ‘on the inside’; we're multiplying $\,x\,$ by $\,3\,$ before dropping it into the $\,f\,$ box. This is a horizontal shrink.

Examples